The Shadows of the Mind

7.Cytoskeletons and Microtubules

If we are to believe that neurons are the only things that control the sophisticated actions of animals,then the humble paramecium presents us with a profound problem. For she swims about her pond with her numerous hairlike legs - the cilia - darting in the direction of bacterial food which she senses using a variety of mechanisms,or retreating at the prospect of danger,ready to swim off in another direction. She can also negotiate obstructions by swimming around them. Moreover,she can apparently even learn from her past experiences - though this most remarkable of her apparent faculties has been disputed by some. How is this all achieved by an animal without a single neuron or synapse? Indeed,being but a single cell,and not being a neuron herself,she has no place to accommodate such accessories.

A paramecium. Note the hair-like cilia that are used for swimming. These form the external extremities of the paramecium's cytoskeleton.

Yet there must indeed be a complicated control system governing the behaviour of a paramecium - or indeed other one-celled animals like amoebas - but it is not a nervous system.The structure responsible is apparently part of what is referred to as the cytoskeleton. As it name suggests,the cytoskeleton provides the framework that holds the cell in shape,but it does much more.The cilia themselves are endings of the cytoskeletal fibres,but the cytoskeleton seems also to contain the control system for the cell,in addition to providing "conveyor belts" for the transporting of various molecules from one place to another.In short,the cytoskeleton appears to play a role for the single cell rather like a combination of skeleton,muscle system,legs,blood circulatory system,and nervous system all rolled into one!

It is the cytoskeleton's role as the cells "nervous system" that will have the main importance for us here.For our own neurons are themselves single cells,and each neuron has its own cytoskeleton! Does this mean that there is a sense in which each individual neuron might itself have something akin to its own "personal nervous system"? This is an intriguing issue,and a number of scientists have been coming round to the view that something of this general nature might actually be true.(See Stuart Hameroff's pioneering 1987 book Ultimate Computing: Biomolecular Consciousness and NanoTechnology;also Hameroff and Wyatt (1982) and numerous articles in the new journal Nanobiology.)

In order to address such issues,we should first glimpse the basic organisation of the cytoskeleton.It consists of protein-like molecules arranged in various types of structure:actin, microtubules, and intermediate filaments.It is the microtubules that will be our main concern here.They consist of hollow cylindrical tubes,some 25nm in diameter on the outside and 14 nm on the  inside (where "nm"= "nanometre", which is 10^-9 m),sometimes organized into larger tubelike fibres that consist of nine doublets,triplets,or partial triplets,of microtubules, organized in an arrangement with a fanlike cross-section,as indicated below,with sometimes a pair of microtubules running down the centre.The paramecium's cilia are structures of this kind.Each microtubule is itself a protein polymer consisting of subunits referred to as tubulin.

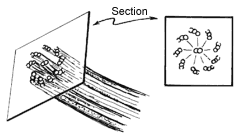Important parts of the cytoskeleton consist of bundles of tiny tubes (microtubules) organized in a structure with a fan-like cross-section. The paramecium's cilia are bundles of this nature.

Each tubulin subunit is a "dimer" ie it consists of two essentially separate parts called a - tubulin and b - tubulin each being composed of about 450 amino acids. It is a globular protein pair,somewhat "peanut shaped" and organized in a slightly skew hexagonal lattice along the entirety of the tube,as indicated below. There are generally 13 columns of tubulin dimers to each microtubule.Each dimer is about 8nm x 4nm x 4nm and its atomic number is about 11 x 10⁴ (which means that it has about that many nucleons in it,so its mass,in absolute units,is about 10^-14).

Each tubulin dimer,as a whole,can exist in (at least) two different geometrical configurations - called conformations.In one of these,they bend to about 30^o to the direction of the microtubule. There is evidence that these two conformations correspond to two different states of the dimer's electric polarization,where these come about because an electron,centrally placed at the a - tubulin /b - tubulin juncture, can shift from one position to the other.

The "control centre" of the cytoskeleton (if indeed this is really an appropriate term) is a structure known as the centriole.This seems to consist essentially of two cylinders of nine triplets of microtubules,where the cylinders form a kind of separated "T"(See below right).

A microtubule. It is a hollow tube,normally consisting of 13 columns of tubulin dimers. Each tubulin molecule is capable of (at least) two conformations.	The centriole (which appears to be the "control centre" of the cytoskeleton-if such exists) consists essentially of a separated "T" built from two bundles of microtubules.

(The cylinders are similar,in a general way,to those that occur in cilia).The centriole forms the critical part of a structure called the microtubules organizing centre or centrosome.Whatever the role of the centriole might be during the normal course of an ordinary cell's existence,it has at least one fundamentally important task. At a critical stage,each of the two cylinders in the centriole grows another,so as to make two centriole "T"s that then separate from each other,each apparently dragging a bundle of microtubules with it - although it would be more accurate to say that each becomes a focal point around which microtubules assemble. These microtubule fibres somehow connect the centriole to the separate DNA strands in the nucleus (at central points,known as their centromeres) and the DNA strands separate - initiate the extraordinary process technically known as mitosis, which simply means cell division (see below).

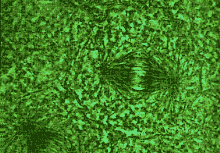In mitosis (cell division) the chromosomes separate, being pulled apart by microtubules.

It may seem odd that there should be two quite different "headquarters" in a single cell.On the one hand there is the nucleus,where the fundamental genetic material of the cell resides,which controls the cell's heredity and its own particular identity,and governs the production of the protein materials of which the cell itself is composed. On the other hand,there is the centrosome with its chief component the centriole,which seems to be the focal point of the cytoskeleton,a structure that apparently controls the cell's movements and its detailed organization. The presence of these two different structures in eukaryotic cells (the cells of all animals and almost all plants on this planet - but excluding bacteria,blue-green algae,and viruses) is believed to be the result of an ancient "infection" that took place some thousands of millions of years ago. The cells that previously inhabited the earth were prokarytoic cells that still exist today as bacteria and blue-green algae,and which possess no cytoskeleton. One suggestion (Sagan 1976) is that some early prokaryotes became entangled with - or,perhaps,"infected by" - some kind of spirochete,an organism that swam with a whiplike tail composed of cytoskeleton proteins. These mutually alien organisms subsequently grew to live permanently together in a symbiotic relationship as single eukaryotic cells.Thus, these "spirochetes" ultimately became cells' cytoskeletons - with al the implications for the future evolution that thereby made us possible!

The organization of mammalian microtubules is interesting from a mathematical point of view. The number 13 might seem to have no particular mathematical significance,but this is not entirely so.It is one of the famous Fibonacci numbers:

0,1,1,2,3,5,8,13,21,34,55,89,144,.....

A sunflower head. As with many other plants,Fibonacci numbers feature strongly. In the outer regions,there are 89 clockwise and 55 anticlockwise spirals. Nearer the center we can find other Fibonacci numbers.

where each successive number is obtained as a sum of the previous two. This might be fortuitous, but Fibonacci numbers are well known to occur frequently (at a much larger scale) in biological systems. For example, in fir cones,sunflower heads,and palm tree trunks,one finds spiral or helical arrangements involving the interpenetration of right-handed and left-handed twists,where the number of rows for one handedness and the number for the other handedness are two successive Fibonacci numbers (see below).(As one examines the structures from one end to the other,one may find that a "shunt" takes place,and the numbers then shift to an adjacent pair of successive Fibonacci numbers.) Curiously,the skew hexagonal pattern of microtubules exhibits a very similar feature - generally of an even more precise organization - and it is apparently found (at least normally) that this pattern is made up of 5 right-handed and 8 left-handed helical arrangements (see below).

	View down a microtubule! The 5 + 8 spiral arrangement of the tubulins in this microtubule can be seen.
	Imagine a microtubule slit along its length, and then opened out flat into a strip. We find that the tubulins are ordered in sloping lines which rejoin at the opposite edge 5 or 8 places displaced (depending upon whether the lines slope to the right or to the left).

In the diagram I have tried to indicate how this structure might appear as actually "viewed" from within a microtubule. The number 13 features here in its role as the sum: 5+8. It is curious,also,that the double microtubules that frequently occur seem normally to have a total of 21 columns of tubulin dimers forming the outside boundary of the composite tube - the next Fibonacci number! (However, one should not get carried away with such considerations; for example,the "9" that occurs in the bundles of microtubules in cilia and centrioles is not a Fibonacci number.) [But it is the square of a Fibonacci number,but if one included all indices of Fibonacci's a great many numbers would be involved increasing the likelihood of some biological entity to have made use of it -LB]

Why do Fibonacci numbers arise in microtubule structure? In the case of fir cones and sunflower heads,etc.,there are various plausible theories - and Alan Turing himself was someone who thought seriously about the subject (Hodges 1983, p437).But it may well be that these theories are not appropriate for microtubules, and different ideas are probably relevant at this level. Koruga (1974) has suggested that these Fibonacci numbers may provide advantages for the microtubule in its capacity as an "information processor".Indeed,Hameroff and his colleagues have argued,for more than a decade,that microtubules may play roles as cellular automata,where complicated signals could be transmitted and processed along the tubes as waves of differing electric polarization states of the tubulins. Recall that tubulin dimers can exist in (at least) two different conformational states that can switch from one to the other,apparently because of alternative possibilities for their electric polarizations. The state of each dimer would be influenced by the polarization states of each of its six neighbours (because of van der Waals interactions between them) giving rise to certain specific rules governing the conformation of each dimer in terms of the conformations of its neighbours. This would allow all kinds of messages to be propagated and processed along the length of each microtubule. These propagating signals appear to be relevant to the way that microtubules transport various molecules alongside them,and to the various interconnections between neighbouring microtubules - in the from of bridge-like connecting proteins referred to as MAPs (microtubule associated proteins) (see below).Koruga argues for a special efficiency in the case of Fibonacci-number-related structure of the kind that is actually observed for microtubules.There must indeed be some good reason for this kind of organization in microtubules,since although there is some variation in the numbers that apply to eukaryotic cells generally,13 columns seems to be almost universal amongst mammalian microtubules.

Microtubules tend to be interconnected with neighbouring ones by bridges of microtubule associated proteins (MAPs).

What is the significance of microtubules for neurons? Each individual neuron has its own cytoskeleton.What is its role? I am sure that there is a great deal to be uncovered by future research,but it seems that already a fair amount is known. In particular,microtubules in neurons can be very long indeed,in comparison with their diameter (which is only about 25-30nm) and can reach lengths of millimetres or more. Moreover,they can grow or shrink,according to circumstances,and transport neurotransmitter molecules. There are microtubules running along the lengths of axons and dendrites. Although single microtubules do not seem to extend individually to the entire length of an axon,they certainly form communicating networks that do so,each microtubule communicating with the next ones by means of the connecting MAPs referred to above. Microtubules seem to be responsible for maintaining the strengths of synapses,and no doubt, for effecting alterations of these strengths when the need arises. Moreover,they seem to organize the growth of new nerve endings,guiding them towards their connections with other nerve cells.

Since neurons do not divide after the brain is fully formed,there is not a role of this particular kind for a centriole in a neuron.Indeed,centrioles seem to be absent in the neuron's centrosome - which is found close to the neuron's nucleus. Microtubules extend from there right up to the vicinity of the vicinity of the presynaptic endings of the axon,and also,in the other direction,into the dendrites and dendritic spines that frequently  form the postsynaptic end of a synaptic cleft. These spines are subject to growth and degeneration, a process which seems to form an important part of brain plasticity,whereby the overall interconnections in the brain are undergoing continual subtle changes. There would seem to be significant evidence that microtubules are indeed importantly involved in the control of brain plasticity.

As an apparent curiosity,it may also be mentioned that in the presynaptic endings of axons there are certain substances associated with microtubules which are fascinating from the geometrical point of view,and which are important in connection with the release of neurotransmitter chemicals. These substances - called clathrins - are built from protein trimers known as clathrin triskelions,which form three-pronged (polypeptide) structures. The clathrin triskelions fit together to make beautiful mathematical configurations that are identical in general organization to the carbon molecules known as "fullerenes" (or "bucky balls") owing to their similarity with the famous geodesic domes constructed by the American architect Buckminster Fuller. Clathrins are much larger than fullerene molecules,however,since an entire clathrin triskelion,a structure involving several amino acids,takes the place of the fullerene's single carbon atom. The particular clathrins that are concerned with the release of neurotransmitter chemicals at synapses seem mainly to have the structure of a truncated icosahedron - which is familiar as the polyhedron demonstrated in the modern soccer ball! 

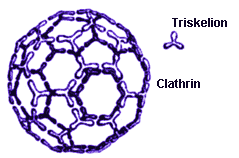A clathrin molecule (similar in overall structure to a fullerene,but made of more complicated substructures - triskelion proteins rather than carbon atoms). The clathrin depicted resembles an ordinary soccer ball in structure.

In the previous section,the important question was raised: what is it that governs the variation in the strengths of synapses and organizes the places where functioning synaptic connections are to be made? We have been guided to a clear belief that it is the cytoskeleton that must play a central role in this process. How does this help us in our quest for a non-computational role for the mind? So far, all we seem to have gained is an enormous potential increase in computing power over and above what could have been achieved if the units were simply the neurons alone.

Indeed,if tubulin dimers are the basic computational units,then we must envisage the possibility of a potential computing power in the brain that vastly exceeds that which has been contemplated in the AI literature. Hans Moravec,in his book Mind Children (1988),assumed,on the basis of a "neuron alone" model,that the human brain might in principle conceivably achieve some 10¹⁴ basic operations per second,but no more,where we consider that there might be some 10¹¹ operational neurons,each capable of sending about 10³ signals per second. If,on the other hand,we consider the tubulin dimer as the basic computational unit,then we must bear in mind that there are some 10⁴ dimers per neuron,the elementary operations now being performed some 10⁶ times faster,giving us a total of around 10²⁴ operations per second,as Moravec and others would strongly argue,there is no prospect of the 10²⁴ figure being achieved in the foreseeable future.

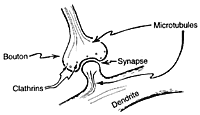Clathrins,like those above (and microtubule endings) inhabit the axon's synaptic boutons and seem to be involved in controlling the strength of the synaptic connection.

Of course it could reasonably be claimed that the brain is operating nowhere remotely close to the 100% microtubular efficiency that these figures assume. Nevertheless, it is clear that the possibility of "microtubular computing" puts a completely different perspective on some of the arguments for imminent human-level artificial intelligence. Can we even trust suggestions that the mental faculties of a nematode worm have already been computationally achieved merely because its neural organization appears to have been mapped and computationally simulated? As remarked earlier the actual capabilities of an ant seem to outstrip by far,anything that has been achieved by the standrad procedures of AI.One might well wonder how much an ant gains from its enormous array of nano-level "microtubular information processors",as opposed to  what it could do if it only had "neuron type switches". As for paramecium,there is no case to answer.

Yet the arguments of Part 1 are making a stronger claim.I am contending that the faculty of human understanding lies beyond any computational scheme whatever. If it is microtubules that control the activity of the brain,then there must be something within the action of microtubules that is different from mere computation. I have argued that such non-computational action must be the result of some reasonably large-scale quantum-coherent phenomenon,coupled in some subtle way to macroscopic behaviour,so that the system is able to take advantage of whatever new physical processes must replace the stop-gap R-procedure of present day physics.As a first step,we must look for a genuine role for quantum coherence in cytoskeletal activity.

MAIN INDEX
REFERENCE GUIDE			TRANSCRIPTS				GLOSSARY
Chaos	Quantum	Logic	Cosmos	Conscious	Belief	Elect.	Art	Chem.	Maths